Standard Deviation Definition - How to Calculate & Use It with Stocks

In statistics, standard deviation (SD) is a unit of measurement that quantifies certain outcomes relative to the average outcome.

Not only does standard deviation help traders quantify certain outcomes, it also shows us that while occurrences may appear random in the short term, the more occurrences we generate, the more consistent our results become. Thousands of occurrences typically start to create a bell curve around the median value.

This idea goes hand in hand with implied volatility (IV) in the stock market, which refers to the implied magnitude, or one standard deviation range, of potential movement away from the stock price in a year's time.

In other words, a low implied volatility environment tells us that the market is not expecting the stock price to move much away from the current stock price. This lack of implied volatility results in a range of outcomes with a narrow standard deviation of the stock near the current stock price.

A high implied volatility environment tells us that the market is expecting the stock price to move away from the current price with a greater magnitude. This high implied volatility results in a range of outcomes with a wide standard deviation away from the stock price.

Finding the standard deviation of a stock can be cumbersome with the complexity of the Black-Scholes model, and these implied ranges are based on annual expected moves.

At tastylive, we use the expected move formula, which allows us to calculate the one standard deviation range of a stock based on the days-to-expiration (DTE) of our option contract, the stock price, and the implied volatility of a stock:

For example, the 1SD expected move of a $100 stock with an IV% of 20% is between +- $20 of the current stock price, or a range between $80 and $120.

Before diving into how it applies to options trading, it’s important to understand the probabilities associated with certain multiples of standard deviations:

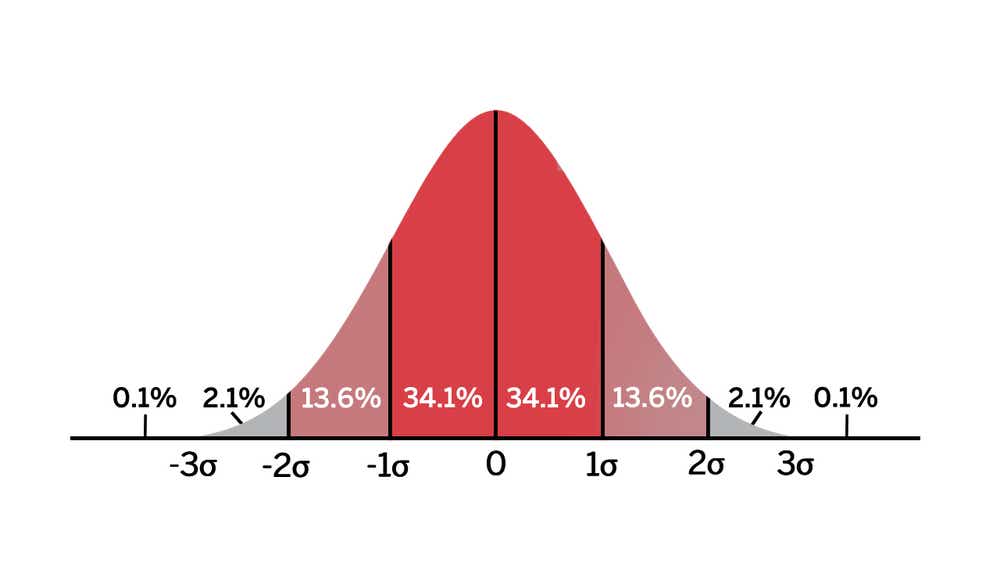
• One standard deviation of a stock encompasses approximately 68.2% of outcomes in a distribution of occurrences based on current implied volatility
• Two standard deviations of a stock encompasses approximately 95.4% of outcomes in a distribution of occurrences based on current implied volatility
• Three standard deviations of a stock encompasses approximately 99.7% of outcomes in a distribution of occurrences based on current implied volatility

Think of any stock you like, and consider tracking how many times in a row it goes up in price, or down in price, for consecutive days. Over a large window of time, you’ll see that the vast majority of stock price movement would land in the one standard deviation range of outcomes.

This may be something like 1-3 days in a row moving in the same direction. Going out to 2 standard deviations would certainly have less occurrences, and would track something like 4-7 days in a row moving in the same direction. 3 standard deviations would encompass the fewest occurrences of 7+ days in a row moving in the same direction.

As you can see, the highest number of occurrences will generally encompass what we expect, and the lowest number of occurrences will encompass outlier events.

The standard deviation of a particular stock can be quantified by examining the implied volatility of the stock’s options. The implied volatility of a stock is synonymous with a one standard deviation range in that stock. Remember, the higher the implied volatility is, the wider our standard deviation range of outcomes is.

This example above may be considered a low implied volatility environment. In a high IV environment, maybe the IV is 40%. This would mean the one standard deviation range is now between $60 and $140. A much wider expected range will always be tied to higher and higher implied volatility values.

Referring to the bell-curve image above, you can see that standard deviation is measured on both sides of the market. If we know that one standard deviation of a stock encompasses approximately 68.2% of outcomes in a distribution of occurrences, based on current implied volatility, we know that 31.8% of outcomes are outside of this range.

In options terms, “outside of the range” equates to the probability of an out-of-the-money (OTM) strike moving in-the-money (ITM). Remember though, this accounts for both sides of the market.

To find the probability for just one side of the market, we need to divide this 31.8% number in half, arriving at roughly 16%.

This is the figure we are looking for when viewing the probability of a strike expiring ITM, on a one standard deviation basis. Alternatively, we can look at the 84% probability of an option expiring OTM, which will land you on the same strike result. Both will give us strikes that encompass the range for roughly 68% of implied occurrences, which is how we get our one standard deviation range.

One cool thing about the standard deviation of a stock & implied volatility is that when IV is high, we can obtain these probabilities using much wider strikes. Implied volatility is high, which means there is a larger implied range that the stock can move. That directly translates to higher probabilities of being ITM for further out strikes from a premium selling perspective, and being able to move strikes even further away from the stock to achieve a 1SD range compared to a low implied volatility environment. That’s the power of high implied volatility, and how it affects our trade entry and proximity from the stock price.

As you can see, understanding what implied volatility is telling you about a stock’s expected future movements is very valuable, and can change our options trading strategy altogether depending on how high or low IV is.

Standard deviation gives us a range of expectations around results. Variance refers to the very random nature of a small cluster of results. The lower our number of occurrences are, the more disconnected the results will be from expectation. Consider the coin flip again - over 10,000 occurrences I would expect the results to be 50/50 heads vs tails. Over just five occurrences though, I would not be surprised to see all of them land on heads. The higher our number of occurrences are, the more our actual results will align with expectations.

In trading, this tells us that even if we have a high probability trade or a strong assumption, we still need to account for the fact that the trade is just one occurrence, and we should prepare for the result to potentially be disconnected from expectation. Over a high number of trades though, we should expect our expected probabilities to align with real results.