How to Capture Interest Rates in SPY

How to Capture Interest Rates in SPY
By:Mike Butler
Higher interest rates have increased the SPDR S&P 500 Trust's risk-free rate of return in certain options strategies. Here is what you need to know.
- Long-term calls in SPY now reflect the risk-free rate of return.
- Strategic implications with options are in play with this new-found call skew.
- 100 long shares of SPY + a synthetic short stock position with options reflects the risk-free rate of return.
A few years ago, interest rates were close to zero, and finding ways to earn interest on idle cash was difficult. Now, with interest rates exceeding 5%, it shines a light on the SPDR S&P 500 Trust (SPY) and how the interest rate is baked into the call options prices.
When you look up the Black-Scholes Model calculation, you quickly realize you're not in for a good time. But you can see the risk-free rate of return is an input into the pricing model for call options. That means that as you look at a SPY options chain, you can see that the farther out in time you go, the more expensive call options are relative to the same-strike put option.
SPY call skew reflects the interest rate
Skew in equidistant options prices can be a result of a few different things, like heightened implied volatility or a big realized move that happened recently. However, in the case of SPY where put skew typically exists, if we're seeing call skew it's likely because of an increased risk-free rate of return, which is reflected in the call options prices:
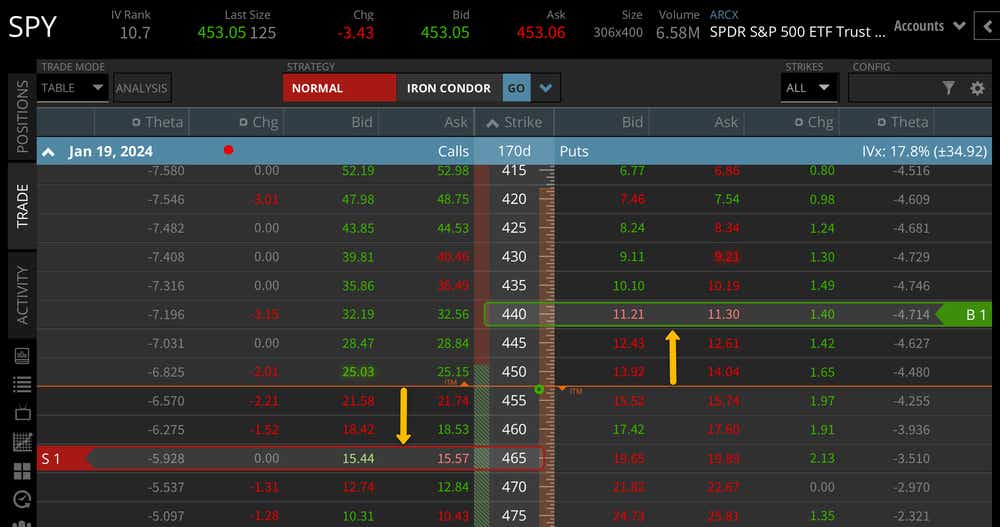
As we can see here, with SPY trading at $453, the 465 strike call is trading for $15.50 and the 440 strike put is trading for around $11.25. Both of these options are about 12-13 points from the current price of SPY, and if there were no skew these options would be trading for the same value. In fact, if you look at more near-term options, you can see this call skew doesn't exist, and in some situations put skew exists, where the put contract is trading for more than the call contract when comparing equidistant out-of-the-money (OTM) options.
Capturing SPY dividends and the interest rate
A classic example is a synthetic short stock position with options, where buying a put and selling a call would typically have zero net extrinsic value and bring your breakeven right to the stock price because it reflects the same risk profile as 100 short shares of stock.
However, if you're looking at long-term options contracts in SPY, you can see this is not the case:
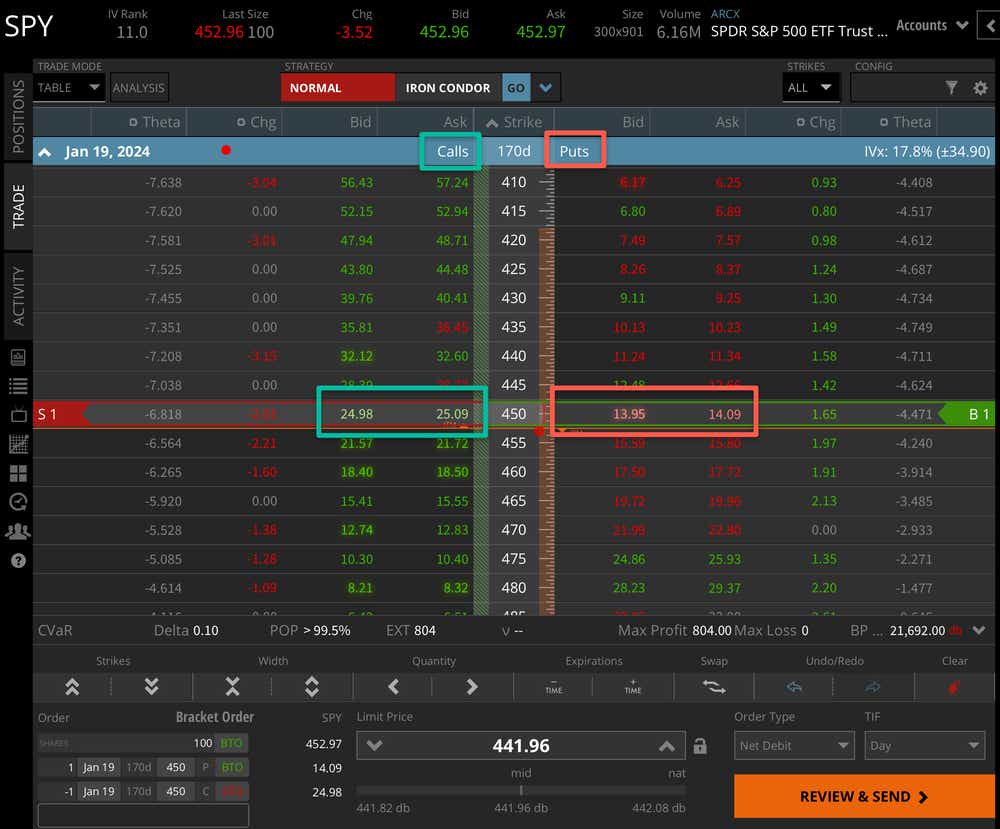
If this short stock position were paired with 100 shares of long stock, there would be no directional risk in the shares, and the positive extrinsic value collected from the short stock position would decay over time, resulting in capturing the interest rate and dividends in SPY, which nets out to the interest rate currently offered in the market. Looking at the image above, the call is trading for about $25.00 and the put is trading for about $14.00, and after removing the intrinsic value of the position we're left with $800 in extrinsic value that would decay over time.
As I stated before, this number can change as the Fed makes decisions on interest rates. For example, if I captured the extrinsic value with a position like this and the Fed cut interest rates, I may see extrinsic value come out of the position quickly. On the other hand, if they hike interest rates, I may see extrinsic value become infused into the position.
With all of this said, there are many ways to strategize around the increased premium in call options due to the increased risk-free rate of return. With a covered call strategy, for example, I'm collecting more than if interest rates were zero.
Overall, it's an interesting time in the markets, where we're fluttering just off of all time highs and yet interest rates are climbing at the same time.
Tune in to Options Trading Concepts LIVE , Monday through Friday at 11 a.m. CDT for more options trading analysis like this!
Mike Butler, tastylive director of market intelligence, has been in the markets and trading for a decade. He appears on Options Trading Concepts Live, airing Monday-Friday. @tradermikeyb
For live daily programming, market news and commentary, visit tastylive or the YouTube channels tastylive (for options traders), and tastyliveTrending for stocks, futures, forex & macro.
Trade with a better broker, open a tastytrade account today. tastylive, Inc. and tastytrade, Inc. are separate but affiliated companies.
Options involve risk and are not suitable for all investors. Please read Characteristics and Risks of Standardized Options before deciding to invest in options.